by Group 7
Theory of Relativity (Simplified)
The Theory of Relativity is a concept created by Albert Einstein, it is composed of 2 theories Special Relativity and General Relativity.
Special Relativity applies to all physical phenomena in the abstence of gravity.
General Relativity explains the Law of Gravitation and its relations to other forces of nature; it aplies to the cosmological and astrophysical real, including astronomy

Special Relativity
In physics, the special theory of relativity, or special relativity for short, is a scientific theory regarding the relationship between space and time. The theory is based on 2 postulates.
-
The laws of physics are invariant (that is, identical) in all inertial frames of reference (that is, frames of reference with no acceleration).
-
The speed of light in vacuum is the same for all observers, regardless of the motion of the light source or observer.
Special relativity was originally proposed by Albert Einstein in a paper published on 26 September 1905, The incompatibility of Newtonian mechanics with Maxwell's equations of electromagnetism and, experimentally, the Michelson-Morley null result demonstrated that the historically hypothesized luminiferous aether did not exist. This led to Einstein's development of special relativity, which corrects mechanics to handle situations involving all motions and especially those at a speed close to that of light.

General Relativity


Event
In physics, and in particular relativity, an event is the instantaneous physical situation or occurrence associated with a point in spacetime.
Upon choosing a frame of reference, one can assign coordinates to the event:
Three spatial coordinates (x)=(x,y,z) to describe the location and one time coordinate (t) to specify the moment at which the event occurs (time). These four coordinates (x,t) together form a four-vector associated with the event.
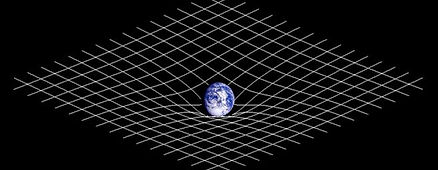
Is the current description of gravitation in modern physics. General relativity generalizes special relativity and refines Newton's law of universal gravitation, providing a unified description of gravity as a geometric property of space and time or four-dimensional spacetime.
In particular, the curvature of spacetime is directly related to the energy and momentum of whatever matter and radiation are present. The relation is specified by the Einstein field equations, a system of partial differential equations.
Einstein's theory has important astrophysical implications. For example, it implies the existence of black holes regions of space in which space and time are distorted in such a way that nothing, not even light, can escape as an end-state for massive stars. There is ample evidence that the intense radiation emitted by certain kinds of astronomical objects is due to black holes. Widely acknowledged as a theory of extraordinary beauty, general relativity has often been described as the most beautiful of all existing physical theories.
Soon after publishing the special theory of relativity in 1905, Einstein started thinking about how to incorporate gravity into his new relativistic framework. In 1907, beginning with a simple thought experiment involving an observer in free fall, he embarked on what would be an eight-year search for a relativistic theory of gravity. After numerous detours and false starts, his work culminated in the presentation to the Prussian Academy of Science in November 1915 of what are now known as the Einstein field equations, which form the core of Einstein's general theory of relativity

Modern Applications
Far from being simply of theoretical interest, relativistic effects are important practical engineering concerns. Satellite-based measurement needs to take into account relativistic effects, as each satellite is in motion relative to an Earth-bound user and is thus in a different frame of reference under the theory of relativity. Global positioning systems such as GPS, GLONASS, and Galileo, must account for all of the relativistic effects, such as the consequences of Earth's gravitational field, in order to work with precision. This is also the case in the high-precision measurement of time. Instruments ranging from electron microscopes to particle accelerators would not work if relativistic considerations were omitted.
